Appendix VI - Lesson 20
Chord Analysis
Notes to Appendix VI - Lesson 20
Lesson 20, Chord Analysis must seem like deja vu to the graduates of Mickey's course in Volume 1. Right now might be a great time to review Lesson 19 of Volume one. In this Lesson 20, Mickey talks about chord analysis and he makes a great point: from time to time, we'll need to invent a chord form to fit our arrangement and it may be the only time in our musical careers that we will use that particular fingering.
That's old hat, actually for those of us that are fingerstyle guitarists. With four available fingers on the right hand we can play any group of notes found on any four of the six strings as a block chord. We sometimes use open strings in chords high on the fretboard to get great effects. We can get some effects that pick stylists only can imagine.
Just why do some chords have different names? If we were renaissance lutenists we wouldn't have that problem. In those days the most complex chord was a triad built of thirds. We needed the Church's permission to play a dominant 7 chord because it had the feared "Devil's interval", the tri-tone or Diminished 5 in it. And the vii triad, which had a naturally occuring tri-tone, was avoided. If you will, picture a lutenist sitting in a courtyard when he happens to finger a G7 chord. He becomes fascinated with it and he sits there strumming away using that chord. When no one from the church comes by to arrest him and have him burned at the stake for heresy, and more importantly, a lightning bolt did not strike him dead, he began to be bolder and experiment with more 7th chords.
As music evolved, we went to 7th chords (4-note chords), then to 9ths (5-note chords), 11ths (6-note chords), and finally to 13ths (7-note chords). We then began to experiment with altering 5ths and 9ths and later 11ths. As long as we are playing all the notes of the chord, it wasn't a problem and the name of the chord would be apparent.
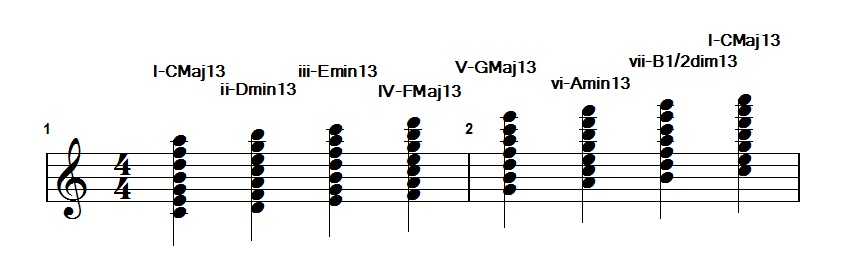
But the guitar only has 6 strings so a full 7-note chord is impossible. We can argue that if we have a bass player, he can play the root, and we can play the other 6 notes and make a 7-note chord that way. Practically speaking, we think more in terms of 3- and 4-note harmonies. We discovered that we can omit the chord's 5th and it does little to affect the sound, as the 5th is the 2nd overtone of the root. And probably the next note we can omit is the root, especially if we have a bass player we can convince to play it from time to time. But these are general rules and they don't always fit the situation. We need to select four notes (assuming we're in 4-part harmony). Let's look at a C Major Scale harmonized to 13th chords (C Maj13, D min13, E min 13, F Maj13, G13, A min13, B diminished13):

C Major Scale Harmonized to 13ths
I chose C Major due to no sharps or flats and none of the chords have any accidentals. A 13 chord uses all the notes of the scale and also, in 13 harmony, there are no inversions. The root of the chord is its name. Let's examine this scale closely (that's why I made it a little bigger!) Remember that we count from the bottom. Four notes up is a 7th chord, five is a 9th, etc.
The first thing you may note is that you can find ANY triad from the C Major Scale in ANY chord. Let's also continue with our self-imposed limitation of 4 notes plus our bass playing a note. Look at the I chord and notice that a C Maj9 has a C, E, G, B, and D. The bassist plays the C and we play the rest. Now notice that the iii chord has E, G, B, and D, an E min7. As long as our bass player plays a C, we can play any E min7 chord form, and our audience hears a C Maj9 chord. A 1st inversion E min7 chord is G, B, D, E, which we also know as a G Maj6 chord. Likewise when the bassist plays a C, we can play also play any chord form of a G6 chord, and our audience hears a C Maj9 chord.
We don't have to use big, colorful chords for this example. How can we forget that Mickey's first substitution for a C Major triad is a C Maj7 to C Maj6. Our bassist plays the C note and we play an E min triad and go to an A min triad, and our audience hears C Maj7 to C Maj6. If we get ambitious and play E min7 and then A min7, our audience hears C Maj9 to C Maj6.
The iii chord in C is an E min. Looking at our scale above we see that an Emin9 as a iii chord in C has as its 9th, an F natural. If we go to the key of G Major, an E min is the vi chord. The 9th of that chord is an F#.
Note that the IVMaj7 is all the notes of a ii9 chord, except the root.
Just when we were about to scrape all of our triad chords for more complex ones, we can see just how useful triads can be. I've included a set of bonus G Major scales harmonized as natural triads and inversions, but we going to play the open 4th string, D as a pedal note. Note how pleasing all of the chords sound. We can deduce from this exercise that we can use any triad from its scale as a passing chord as long as it is between our two chords.
Read through Mickey's examples using the above logic and you won't have any trouble understanding how a chord can have two names. But what name do you use? My personal rule is that I try to first use the name of a chord from the Circle of 4ths, such as C to F to Bb to Eb, etc. If that doesn't work, then I try to use a name from parallel movement , such as D min7 to Db min7 to C min7 to B min7, etc.
If you still can't come up with a name that you think fits the situation, call it whatever you wish with an open conscience, as there is no Chord Police and your name is as good as anyone's.
Let's "comp" that fun!!